NS3 SIMULATOR PROJECT TITLE
Quantizer Design for Distributed GLRT Detection of Weak Signal in Wireless Sensor Networks
We consider the problem of distributed detection of a mean parameter corrupted by Gaussian noise inwireless sensor networks, where a large number of sensor nodes jointly detect the presence of a weak unknown signal. To circumvent power/bandwidth constraints, a multilevel quantizer is employed in each sensor to quantize the original observation. The quantized data are transmitted through binary symmetric channels to a fusion center where a generalized likelihood ratio test (GLRT) detector is employed to perform a global decision.
The asymptotic performance analysis of the multibit GLRT detector is provided, showing that the detection probability is monotonically increasing with respect to the Fisher information (FI) of the unknown signal parameter. We propose a quantizer design approach by maximizing the FI with respect to the quantization thresholds. Since the FI is a nonlinear and nonconvex function of the quantization thresholds, we employ the particle swarm optimization algorithm for FI maximization. Numerical results demonstrate that with 2- or 3-bit quantization, the GLRT detector can provide detection performance very close to that of the unquantized GLRT detector, which uses the original observations without quantization.

 Click Here to watch our latest output video using NS3 simulator
Click Here to watch our latest output video using NS3 simulator 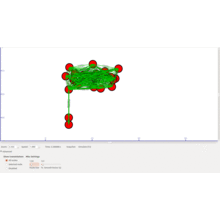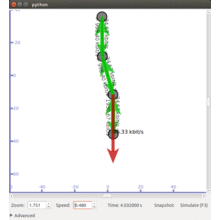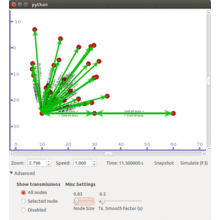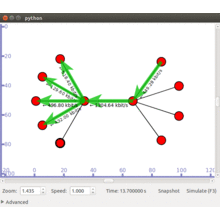 Click Here to watch our latest projects screenshots using NS3 simulator
Click Here to watch our latest projects screenshots using NS3 simulator